On Hyers-Ulam Stability of Non-homogeneous Nonlinear Second Order Differential Equations

Fakunle I.;¹ , Arawomo P.O.;² , Idiong U.S.;³ , Akinremi B.V.;³ & Famuagun K.S.³

¹Adeyemi Federal University of Education, Ondo State, Nigeria

*Corresponding Author Email: fakunlei@aceondo.edu.ng …

Read More

Highlights

Abstract

In this paper, Hyers-Ulam stability of non-homogeneous nonlinear second order differential equations are consid- ered. Conditions are stated to transform nonlinear second order differential equations to integral inequalities for easy application of  Gronwall-Bellman-Bihari  inequality.  Our  results  improved and extended some known results in the literature.  

Keywords and phrases: Nonlinear differential equation, integral inequality, Hyers-Ulam stability, Gronwall-Bellman-Bihari inequality.

1. Introduction

The purpose of this paper is to study Hyers-Ulam stability of the following equations:

and

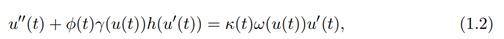
for all t > 0, with initial conditions

Where g:[0,T]×〖R^n⟶R〗^(n×m)is the diffusion function. The noise in equation (1.1) is generally called Gaussian white noise. It is expressed as , where is the Wiener process. For the properties of Wiener process see Higham (2001) and Williams (2006) in Ganiyu et al (2015).
Equation (1.1) can be written as

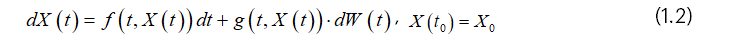
Integrating (1.2) from 0 to t we have

where a(t), b(t), κ(t), φ(t), g(t) ∈ C(R₊), f(u), γ(u), ω(u), h(u) ∈ C(R₊,R₊).

The study of stability for various functional equations originated from a famous talk of Ulam, 1960 who posed a problem concerning the stability of functional equations:” Give conditions in order for a linear function near an approximately linear function to exist”. Since then, this question has attracted the attention of many researchers. Note that the solution to this question was given by Hyers, 1941 for additive functions defined on Banach space in 1941. Thereafter, the result by Hyers 1941, was generalised by Rassias 1978, Aoki, 1950 and Bourgin, 1988. After that, many authors extended the Ulam problem to other functional equations in various directions. In 1998, Alsina and Ger investigated the Hyers-Ulam stability of a differential equation. They have proved that for every differentiable mapping f:I→R satisfying |f(x)-f(x)| ≤∈ for every x∈I I, where ∈ > 0 is a given number and I is an open interval of R, there exists a differentiable function g:I→R with property g^‘ (x)=g(x) and |f(x)-g(x)| ≤ 3∈ for all x∈I. The result of Alsina and Ger, 1998 was extended by Miura et al., 2003a; Miura et al., 2003b , Takahasi et al., 2002, and by Takahasi, et al., 2004 to the Hyers-Ulam stability of the first order linear differential equations and linear differential equations of higher order with constant coefficients. Furthermore, Jung, 2006a; Jung, 2006b; Jung, 2004; Jung 2005 has obtained result on the stability of linear differential equations extending the results of Takahash et al., 2004. Rus, 2010a; Rus, 2010b has proved some results on the stability of linear differential and integral equations using Gronwall’s lemma and the technique of weakly Picard operators.

Recently, Wang, et al., 2008 and Li & Shen 2010 proved the Hyers-Ulam stability of the linear differential equations of the first order and the linear differential equations of the second order with constant coefficients by using the method of integral factor.

Many of the authors prefer to consider the Hyers-Ulam stability of linear differential equations due to the fact that it can easily be handled. In this paper, equations (1.1) and (1.2) will be transformed to integral inequalities using the conditions that we shall prescribe for application of Gronwall-Bellman-Bihari inequality to study the Hyers- Ulam stability. The results established in this paper through Gronwall-Bellman-Bihari inequality extended some of the results in the literature.

2. Preliminary

First of all, we give some definitions, lemmas and theorems which are going to assist us in this work.

2.1.1 Definition 1

We say that equation (1.1) has the Hyers-Ulam stability, if there exists a constant K₁^*≥0 with the following property:  for every ϵ > 0, u(t) ∈ C²(R₊), if

then, there exists some u₀(t) ∈ C²(R₊) such that

|u(t) − u₀(t)| ≤ K₁^∗ϵ.

We call such K₁^* a Hyers-Ulam constant.

2.1.2 Definition 2

Equation (1.2) is Hyers-Ulam stable, if given ϵ > 0 and there exists a solution u(t) ∈ C²(R₊), such that

|u^jj(t) + φ(t)γ(u(t))h(u^j(t)) − κ(t)ω(u(t))u^j(t)| ≤ ϵ,) (2.2)

in addition, there exists positive K₂^* and any solution u₀ (t)∈C² R₊ of the (1.2) with initial condition (1.3) such that
|u(t) − u₀(t)| ≤ K₂^∗ϵ,

We call such K₂^∗ Hyers-Ulam constant for the differential equation (1.2).

2.1.3 Definition 3

A function ω: [0, ∞) → [0, ∞) is said to belong to a class Ψ
if

1. ω(u) is nondecreasing and continuous for u ≥ 0
2. ω(u) ≤ ω(v/u)for all u and v ≥ 1
3. there exists a function φ, continuous on [0, ∞) with ω(αu) ≤ φ(α)ω(u) for α ≥ 0

2.2 Lemma 1:  Bihari, 1957.

Let u(t), f(t) be positive continuous functions defined on a ≤ t ≤ b,(≤ ∞) and K > 0, M ≥ 0, further let ω(u) be a nonnegative nondecreasing continuous function for u ≥ 0, then the inequality

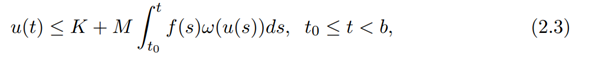
implies the inequality

Where

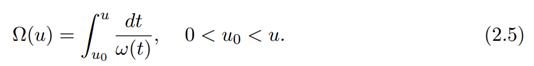
In the case ω(0) > 0 or Ω(0+) is finite, one may take u₀ = 0 and Ω⁻¹ is the inverse function of Ω and t must be in the subinterval [t₀, b₀ ] of [t₀, b] such that

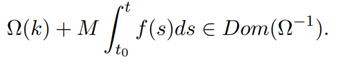
2.2.1 Theorem 1: (Murray, 1974)

If f(t) and g(t) are continuous in [t0, t] ⊆ I and f(t) does not change sign in the interval, then there is a point ξ ∈ [t0, t] such that

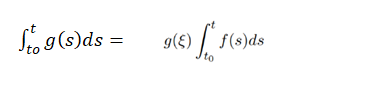
2.2.2. Theorem 2: Fakunle and Arawomo, 2018a; Fakunle and Arawomo, 2018b; Fakunle and Arawomo, 2022a; Fakunle and Arawomo, 2022b.

Suppose u(t), r(t), h(t) ∈ C(I, R+) and $(u), β(u) ∈ Ψ be nonnegative, monotonic, nondecreasing, continuous and ω(u) be a submultiplicative for u > 0.

Let

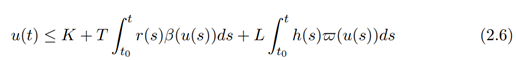
for K, T and L positive constants, then

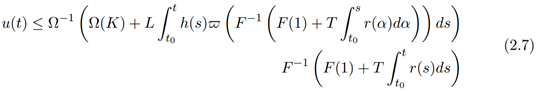
where β(u) 6= $(u), Ω is defined in equation (2.5) and F(u) is defined as

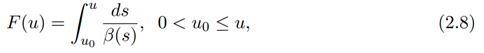
Where F⁻¹, Ω⁻¹ are the inverses of F, Ω respectively and t is in the subinterval (0, b) ∈ I so that

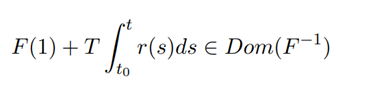
and

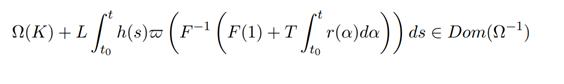
3. Main Results

In this section, we establish the Hyers-Ulam stability of the nonlinear differential equations (1.1), (1.2) as follow: Theorem 3. Assume the following conditions

are satisfied and γ, α ∈ C(R+). In addition, let f, ω ∈ Ψ be continuous, nondecreasing and monotonic, then equation (1.1) has the Hyers-Ulam stability and Hyers-Ulam constant is given as

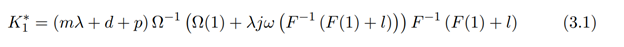
Proof
Evaluating the inequality (2.1) we have

It is clear that

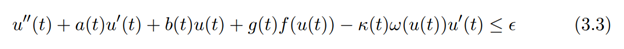
Let

We obtain

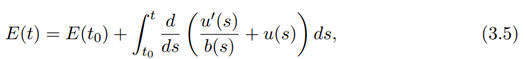
Using equation (1.3), we have

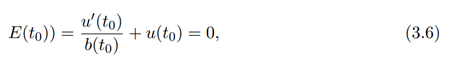
It follows from equation (3.5) that

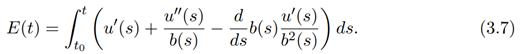
Since b(t) is an increasing function, then d dsb(t) ≥ 0, equation (3.7) reduced to

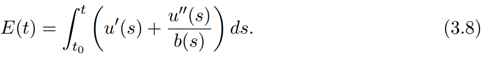
Substituting for u 00(t) in equation (3.8) by using equation (3.3), we have

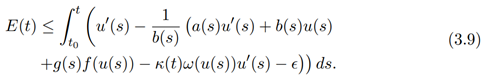
Simplifying equation (3.9), we obtain

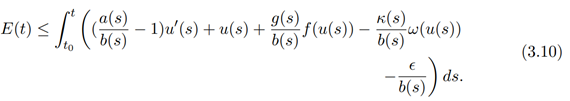
It is clear from equation (3.4) that

Using inequality (3.11) in inequality (3.10), we have

Expressing equation (3.12) further, we obtain

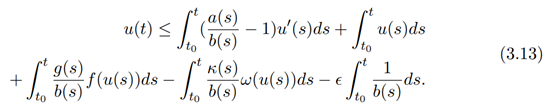
Taking the absolute value of both sides, we have

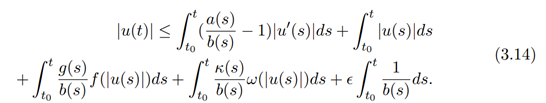
It follows from (3.14) that

Using the conditions (i-v), we get

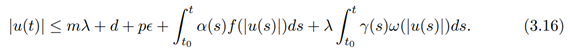
Simplifying equation (3.16) further we have

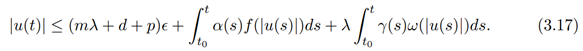
Applying Theorem 2, we obtain

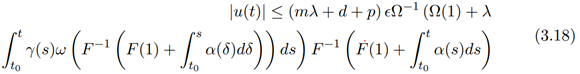
Applying the conditions (vi)- (vii), we arrive at

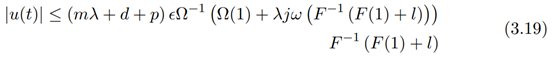
Hence,

Therefore,

3.1.1 Theorem 4

Let u(t) ∈ C 2 (I, R+) satisfies the differential inequality (2.2) for all t ∈ I and for some > 0, then there exists a solution u0(t) ∈ C 2 (I, R+) of equation (1.2) such that

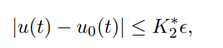
provided the following conditions are satisfied:

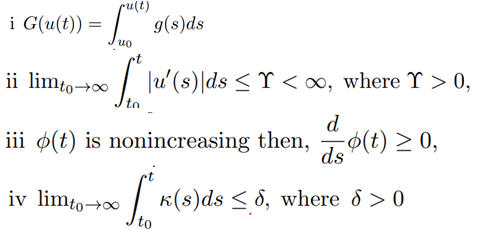
Proof
From equation (2.2), we get

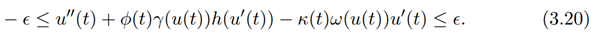
It is clear that

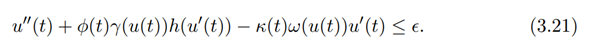
Multiplying equation (3.21) by u 0 (t) and integrating from t₀ to t, we obtain

Using equation (1.3), we get

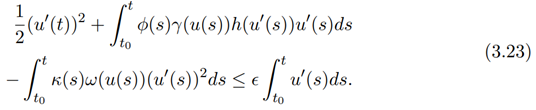
By Theorem 1 there exists ι, µ ∈ [t0, t] such that

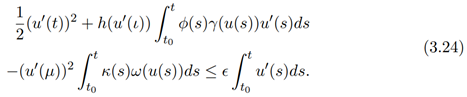
By condition (i) we obtain

Integrating by part using condition (iii) we have

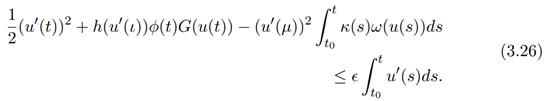
Put

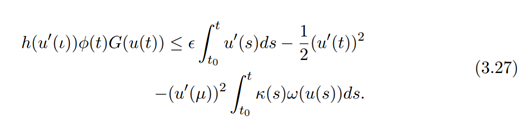
Taking the absolute value of both sides, we have

Using condition (iii) of Theorem 3 and condition (ii) of Theorem 4, we get

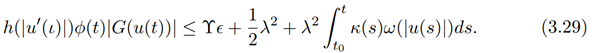
Setting h(|u 0 (ι)|)φ(t)|G(u(t))| ≥ |u(t)|, we obtain

Applying Theorem 1

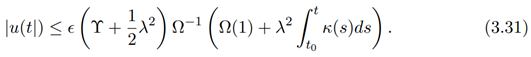
Using the condition (iv), we obtain

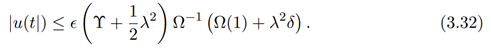
Hence,

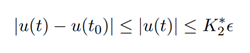
Therefore,

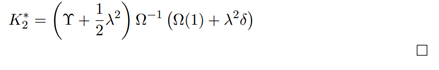
Example 1:

Consider the following equation

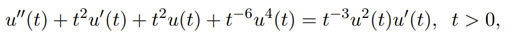
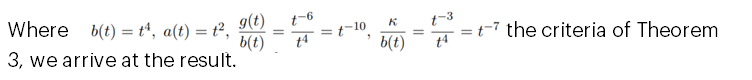
 Example 2:

Consider the following equation

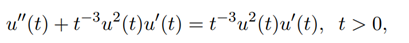
Using the criteria of Theorem 4, we arrive at the result.

Acknowledgement

I acknowledged all reviewers who have been appointed to review this paper.

References

Abdollahpour, A., & Najati, A. (2011). Stability of linear differential equations of third order. Applied Mathematics Letters, 24, 1827-1830.

Alsina, C., & Ger, R. (1988). On some inequalities and stability results related to the exponential function. Journal of Inequalities and Applications, 2, 373-380.

Aoki, T. (1950). On the stability of the linear transformation in Banach spaces. Journal of Mathematical Society of Japan, 2, 64-66.

Bihari, I. (1957). Researches of the boundedness and stability of the solutions of nonlinear differential equations. Acta Mathematica Academiae Scientiarum Hungaricae, 7, 278-291.

Bourgin, D. G. (1988). Classes of transformations and bordering transformations. Bulletin of the American Mathematical Society, 57, 223-237.

Fakunle, I., & Arawomo, P. O. (2018a). Hyers-Ulam stability of certain class of nonlinear second order differential equations. International Journal of Pure and Applied Mathematical Sciences, 11(1), 55-65.

Fakunle, I., & Arawomo, P. O. (2018b). On Hyers-Ulam stability of nonlinear second order ordinary and functional differential equations. International Journal of Differential Equations and Applications, 17(1), 77-88.

Fakunle, I., & Arawomo, P. O. (2019). Hyers-Ulam stability of a perturbed generalised Lienard equation. International Journal of Applied Mathematics, 32(3), 479-489.

Fakunle, I., & Arawomo, P. O. (2022a). Hyers-Ulam-Rassias stability of a certain perturbed nonlinear Lienard type differential equation. To appear in IAENG International Journal of Applied Mathematics.

Fakunle, I., & Arawomo, P. O. (2022b). Hyers-Ulam-Rassias stability of nonlinear second order of a perturbed ordinary differential equation. To appear in Proyecciones Journal of Mathematics.

Hyers, D. H. (1941). On the stability of the linear functional equation. Proceedings of the National Academy of Science of the United States of America, 27, 222-224.

Jung, S.-M. (2004). Hyers-Ulam stability of linear differential equations of first order. Applied Mathematics Letters, 17, 1135-1140.

Jung, S.-M. (2005). Hyers-Ulam stability of linear differential equations of first order (III). Journal of Mathematical Analysis and Applications, 311, 139-146.

Jung, S.-M. (2006a). Hyers-Ulam stability of linear differential equations of first order (II). Applied Mathematics Letters, 19, 854-858.

Jung, S.-M. (2006b). Hyers-Ulam stability of a system of first order linear differential equations with constant coefficient. Journal of Mathematical Analysis and Applications, 320, 554-561.

Li, Y., & Shen, Y. (2010). Hyers-Ulam stability of linear differential equation of second order. Applied Mathematics Letters, 23, 306-309.

Miura, T., Miyajima, S., & Takahasi, S. E. (2003a). A characterisation of Hyers-Ulam stability of first order linear differential operators. Journal of Mathematical Analysis and Applications, 286, 136-146.

Miura, T., Miyajima, S., & Takahasi, S. E. (2003b). Hyers-Ulam stability of linear order differential operators with constant coefficient. Journal of Mathematical Analysis and Numerical Methods, 258, 90-96.

Rassias, T. H. M. (1978). On the stability of the linear mapping in Banach spaces. Proceedings of the American Mathematical Society, 72(2), 297-300.

Rus, I. A. (2010). Ulam stability of ordinary differential equation. Studia Universitatis Babes-Bolyai Mathematica, 54(4), 306-309.

Rus, I. A. (2010). Ulam stability of ordinary differential equations in a Banach space. Carpathian Journal of Mathematics, 126, 103-107.

Schum, R. S. M. (1974). Outline of theory and problems of calculus (SI Metric Edition, International ed.).

Takahasi, S. E., Miura, T., & Miyajima, S. (2002). On the Hyers-Ulam stability of the Banach space-valued differential equation y’ = λy. Bulletin of the Korean Mathematical Society, 39(2), 309-315.

Takahasi, S. E., Takagi, H., Miura, T., & Miyajima, S. (2004). The Hyers-Ulam stability constants of first order linear differential operators. Journal of Analysis and Applied Mathematics, 296, 403-409.

Ulam, S. M. (1960). Problems in modern mathematics (Science Editions, Wily, New York, NY, USA, Chapter 6).

Wang, G., Zhou, M., & Sun, L. (2008). Hyers-Ulam stability of linear differential equations of first order. Applied Mathematics Letters, 21, 1024-1028.

About this Article

Cite this Article

APA

Fakunle I., Arawomo P.O., Idiong U.S., Akinremi B.V. and Famuagun K.S. (2024). On Hyers-Ulam Stability of Non-homogeneous Nonlinear Second Order Differential Equations. In K. S. Adegbie, A. A. Akinsemolu, & B. N. Akintewe (Eds.), Exploring STEM frontiers: A festschrift in honour of Dr. F. O. Balogun. SustainE.

Chicago

Fakunle I., Arawomo P.O., Idiong U.S., Akinremi B.V. and Famuagun K.S. 2024. “On Hyers-Ulam Stability of Non-homogeneous Nonlinear Second Order Differential Equations.” In Exploring STEM Frontiers: A Festschrift in Honour of Dr. F.O. Balogun, edited by Adegbie K.S., Akinsemolu A.A., and Akintewe B.N., SustainE.

Corresponding Author Email: famuagunks@aceondo.edu.ng

Disclaimer: The opinions and statements expressed in this article are the authors’ sole responsibility and do not necessarily reflect the viewpoints of their affiliated organizations, the publisher, the hosted journal, the editors, or the reviewers. Furthermore, any product evaluated in this article or claims made by its manufacturer are not guaranteed or endorsed by the publisher.

Distributed under Creative Commons CC-BY 4.0

Share this article

Use the buttons below to share the article on desired platforms.